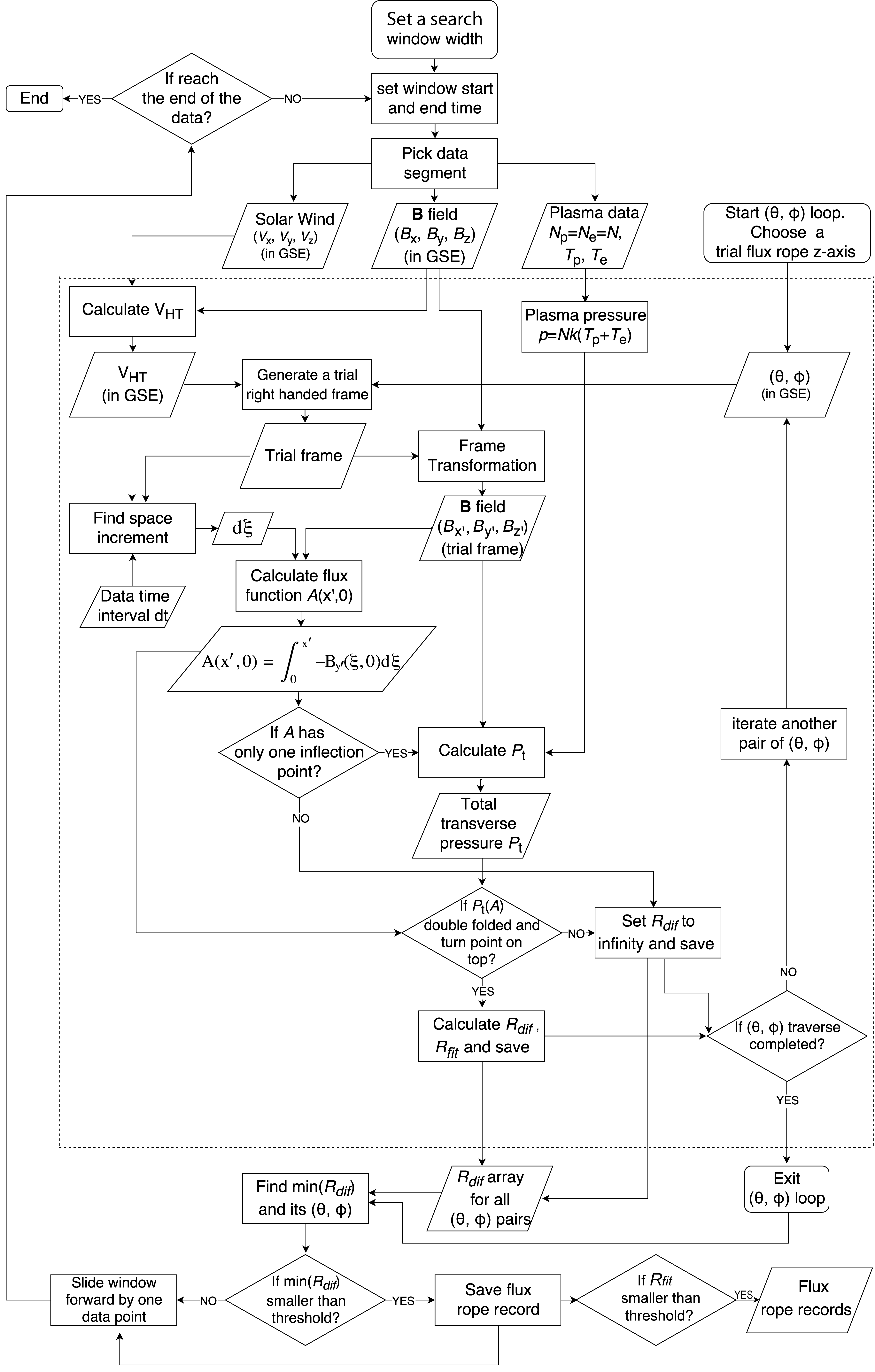
Following Zheng and Hu [2018], the automated detection of small-scale magnetic flux ropes is implemented. Since the standard Grad-Shafranov equation guarantees that the transverse pressure Pt be a single-variable function of the magnetic flux function A, characterizing the cross section of a flux rope [Hu and Sonnerup, 2001, 2002] in this study, the detection of flux ropes can be achieved by evaluating the double-folding pattern of the Pt versus A curve. First, we use multiple sliding windows of variable widths to search for possible flux ropes with different duration ranging from 9 minutes to about 6 hours. During the searching process, all spacecraft measurements are transformed to the quasi-static frame, i.e., usually the deHoffmann-Teller (HT) frame, in order to utilize the Grad-Shafranov equation which is based on the assumption of two-dimensional (2D) magnetohydrostatic equilibrium. After finding the new frame, we spilt the spacecraft path through a flux rope interval into two branches by determining the inflection point of the rotating field component. Values of Pt and A are calculated and two Pt versus A branches are compared to check if there exists double-folding pattern. The difference residue and the fitting residue are introduced to check the quality of this pattern. In addition, the Walén slope, representing the ratio of the remaining flow velocity to the local Alfvén velocity, is used to exclude the strong Alfvénic structures or waves. Lastly, the minimum value of average magnetic field magnitude is set at 5 nT to eliminate events contaminated by small fluctuations in the solar wind. A flowchart illustrating the main procedures in the automated search algorithm is shown above and is described in detail by Hu et al., [2018].